Linkedin Facebook Instagram Youtube

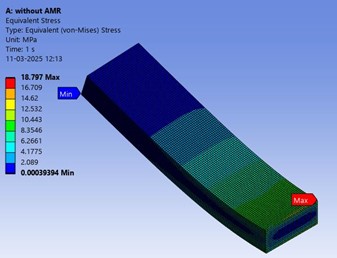
Unlocking Precision: Addressing Stress Singularities Through Local Mesh Refinement

Stress singularities

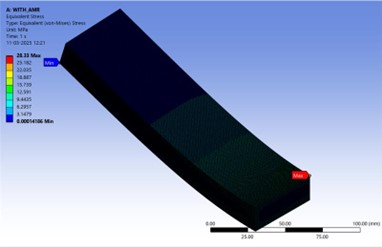
In engineering simulations, stress singularities pose a significant challenge. These occur in areas of a model, such as sharp corners or notches, where stress values can become infinitely large, leading to unreliable results. Addressing these singularities is crucial for creating accurate and safe designs.

One of the most effective ways to mitigate this issue is through local mesh refinement. By increasing mesh density specifically in high-stress regions, we can capture stress behaviour more precisely and avoid the exaggerated stress peaks that typically arise near singularities. In this post, we’ll explore how mesh refinement works, its role in overcoming stress singularities, and best practices for implementing it in your simulations.

Stress singularities

Understanding “Stress Singularities”

Stress singularities occur at points in a model, like sharp corners or notches, were stress values approach infinity. While these extreme stresses don’t happen, they can appear in simulations due to idealized geometry or material properties. These singularities can distort simulation results, leading to inaccurate predictions about material behaviour and failure points. Effectively managing them is crucial to ensure realistic and reliable insights for design and safety-critical applications.

Local Mesh Refinement: A Key Solution

Local mesh refinement is a technique used in finite element analysis (FEA) to improve the accuracy of simulations, particularly in regions with complex stress behaviour. Instead of refining the entire mesh, which can be computationally expensive, this approach focuses on areas that are critical—like sharp edges, holes, or places with high stress gradients.

By increasing the mesh density in these specific regions, local mesh refinement provides a more detailed representation of stress distribution, allowing for more accurate results in areas prone to stress concentrations. This helps in capturing the behaviour of materials under stress more precisely and prevents the unrealistic stress peaks caused by singularities.
The best part is that you can now achieve higher accurate results without significantly increasing the computational cost, as only the critical areas are refined.

Best Practices for Efficient Mesh Refinement

When applying mesh refinement, it’s crucial to focus on the right areas to balance accuracy and computational efficiency. Here are a few best practices:
  • 1. Identify Critical Regions:
    Focus on areas where stress concentrations are most likely, such as sharp corners, notches, and areas with significant geometry changes. These are typically the locations where stress singularities occur and where finer meshes will have the most impact.
  • 2. Use Adaptive Mesh Refinement:
    Adaptive mesh refinement (AMR) automatically adjusts the mesh density based on stress gradients in the model. This allows the mesh to refine in regions with high stress concentrations while maintaining a coarser mesh elsewhere, optimizing computational resources.
  • 3. Ensure Mesh Convergence:
    Perform mesh convergence studies to ensure the results stabilize with finer mesh densities. This helps identify the optimal mesh density where results no longer change significantly, saving time and computational effort.
  • 4. Avoid Over-refinement:
    While refining the mesh can improve accuracy, over-refining can lead to unnecessarily high computational costs. Always ensure that the benefits of refinement outweigh the increased time and memory requirements.
  • 5. Leverage Ansys Features via CADFEM:
    CADFEM provides access to advanced meshing and simulation capabilities through Ansys, which enables precise control over local mesh refinement. These features help streamline the process of optimizing your simulations while balancing accuracy with computational efficiency.

Practical Example: How CADFEM Solves the Challenge

Consider a simulation of a bracket with a sharp corner, where stress concentrations are expected. Without mesh refinement, the simulation might predict unrealistic stress peaks at the corner, leading to incorrect conclusions about the bracket’s strength.
By applying local mesh refinement in this critical area using Ansys, the mesh density is increased around the corner, allowing for a more accurate stress distribution. The results show a more realistic stress concentration that closely matches what would be expected in real-world conditions, ensuring the design’s reliability.
With CADFEM’s expertise in integrating Ansys tools, this refinement process is streamlined, enabling engineers to make informed design decisions while minimizing computational costs.

Conclusion

Stress singularities are a common challenge in finite element simulations, often leading to unrealistic results in areas like sharp corners or notches. Local mesh refinement offers an effective solution by increasing mesh density in critical regions, providing more accurate stress predictions and mitigating the impact of these singularities.

By identifying key stress-concentrated areas, using adaptive meshing, and ensuring mesh convergence, engineers can balance accuracy and computational efficiency. Tools like Ansys, available through CADFEM, make this process more streamlined, allowing for better design insights and more reliable simulations.

© Copyright 2025 by CADFEM APAC

Legal Notices

Privacy Policy

Terms of Use

Cookie Policy

Export Control Regulations

Terms and Conditions

Site Map